Given: Steady flow
- \( T_{1}=30^{\circ}\text{C}=303\,\text{K} \), \( p_{1}=150\,\text{kPa} \), \( D_{1}= ⌀5\,\text{cm} \), \( V_{1}=100\,\text{m/s} \) (Inlet)
- \( T_{2}=30^{\circ}\text{C}=303\,\text{K} \), \( p_{2}=75\,\text{kPa} \), \( D_{2}= ⌀1.5\,\text{cm} \), \( V_{2}=150\,\text{m/s} \) (Inlet)
- \( T_{3}=30^{\circ}\text{C}=303\,\text{K} \), \( p_{3}=90\,\text{kPa}, D_{3}= ⌀9\,\text{cm} \), \( V_{3}=?\,\text{m/s} \) (Outlet)

The schematic diagram of the problem description is shown in Fig. 1.

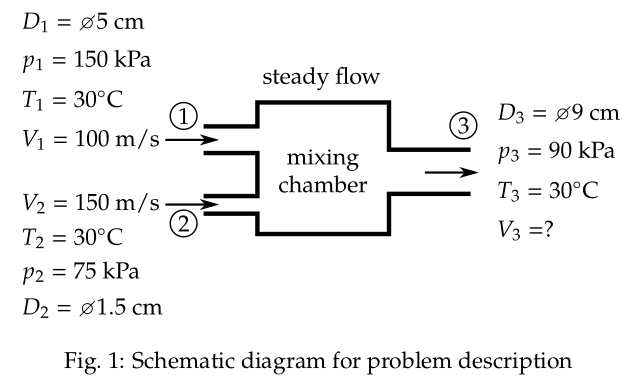
The densities of air at the various stations may be calculated using the respective pressures, temperatures and using the ideal gas equation.

$$ \rho_{1}=\frac{p_{1}}{R\,T_{1}}=\frac{150\times10^{3}}{287\times303}=1.7249\,\text{kg/m}^{3} $$ $$ \rho_{2}=\frac{p_{2}}{R\,T_{2}}=\frac{75\times10^{3}}{287\times303}=0.86246\,\text{kg/m}^{3} $$ $$ \rho_{3}=\frac{p_{1}}{R\,T_{1}}=\frac{90\times10^{3}}{287\times303}=1.0349\,\text{kg/m}^{3} $$

Using the conservation of mass equation,

$$\begin{aligned} m_{1}+m_{2} &= m_{3} \\ \\ \rho_{1}\,A_{1}\,V_{1}+\rho_{2}\,A_{2}\,V_{2} &= \rho_{3}\,A_{3}\,V_{3} \\ \\ \rho_{1}\times\frac{\pi}{4}D_{1}^{2}\times V_{1} + \rho_{2}\times\frac{\pi}{4}D_{2}^{2}\times V_{2} &= \rho_{3}\times\frac{\pi}{4}D_{3}^{2}\times V_{3} \\ \\ 1.7249\times\frac{\pi}{4}\times0.05^{2}\times100 +0.86246\times\frac{\pi}{4}\times0.015^{2}\times150 & = 1.0349\times\frac{\pi}{4}\times0.09^{2}\times V_{3} \end{aligned}$$ $$ V_{3}=\frac{1.7249\times\frac{\pi}{4}\times0.05^{2}\times100 + 0.86246\times\frac{\pi}{4}\times0.015^{2}\times150}{1.0349\times\frac{\pi}{4} \times0.09^{2}} $$ $$ \boxed{V_{3}=54.915\,\text{m/s}}\ . $$